I'm not quite sure why I have always been fascinated by fractals. In some ways, to a mathematically challenged person such as myself, it feels that the enormity of the images allows for an infinite expansion of possibilities into the tiniest details -- a metaphor for the depth and complexity of life.
Or it could be that they remind me of some of my experiences with entheogens -- which also seemed to turn the ordinary into an endlessly complex and evolving tapestry of possibilities.
Wikipedia also has a page on fractal art for those who are interested.
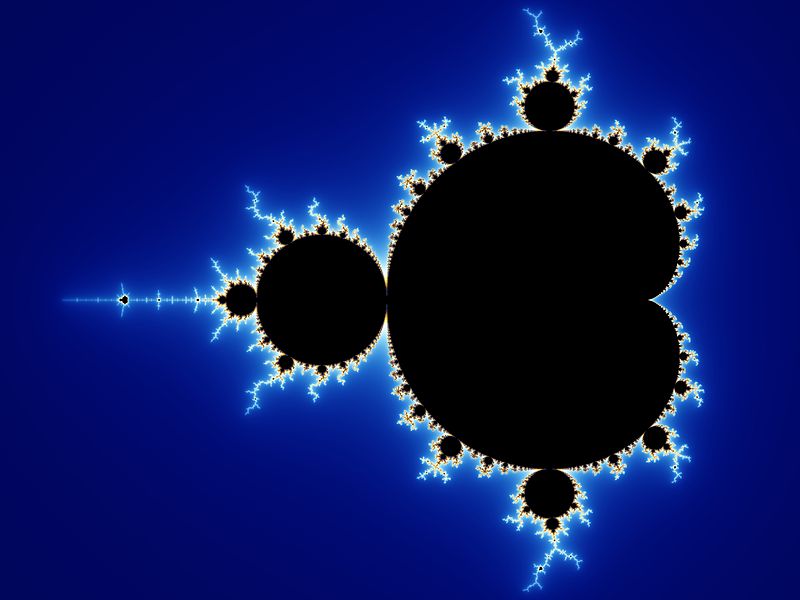

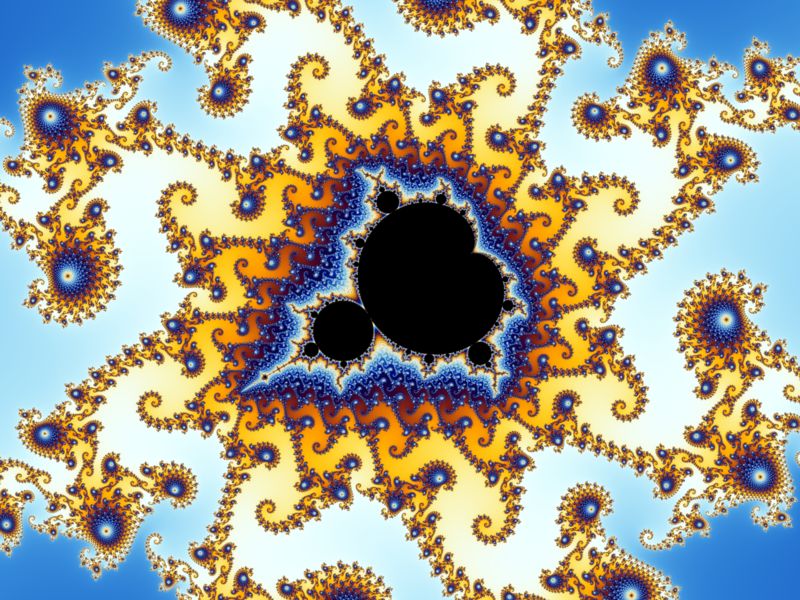


Tags:
5 comments:
Those are great fractal images! And thanks for mentioning the entheogen connection. I have been amazed, but not suprised, by the number of integrally-informed people I have come across that have engaged in consciousness exploration using entheogens and empathogens and have had mind-blowing illuminati experiences (states) as a result.
Take a look at XaoS if you want to explore the Mandelbrot Set yourself!
http://wmi.math.u-szeged.hu/xaos/doku.php?id=main
Fun.
Of course, fractals, despite the origin of the word, are an excellent metaphor for integration via the "as above, so below" idea. Indeed, they also seem to mirror holography. Taking the Mandelbrot set as an example, one discovers the appearance of 'minibrots' (picture #3) at many levels of zoom, each never quite a perfect copy of the whole set, yet recognisably the same. And the set is completely connected, often by threads of infinitessimal breadth. Amazing to think that the whole infinitely complex and beautiful structure is described by the simple iterative mapping (my own descriptor) of z -> z squared + c.
Definitely concur on the entheogen connection: at the height of every such experience, I've 'seen' great vistas of fractal patterns and structures. Some scientists are now saying the universe 'is' a fractal. I have a hunch that those who respond positively to fractals have a natural connection to a very fundamental aspect of the universe, perhaps something beyond the merely manifest.
bill,
thought you might like this discussion of fractals. scroll down 1/3 of the page for Mandelbrot discussion
By Arnold Keyserling and R.C.L.
http://www.schoolofwisdom.com/ChanceandChoice/chapter2.html
The images in that Wiki article are cool, aren't they? Fractal images remdind me of the visions from entheogens as well, or so I imagine. :)
I tried my hand at duplicating those fractal images from the Wiki article on the Mandelbrot set, and think I did a pretty good job. I then went one step further and rendered some of the images as 3D. You can see the results at the link below: (I don't know how to post pictures or inline links to this blog)
http://www.pbase.com/duncanc/wiki_mandel_images
Post a Comment